
How do we measure things that are too big for our measuring tools? Find out how math can help! Get outdoors and harness the power of ratios and geometry to estimate the height of trees and other tall objects.
Age: 10+
Time: 20-30 minutes
Topics: trees, height, measure, estimating
What you need:
- Tree or other tall object, like a telephone pole or building
- Measuring stick or measuring tape
- Chalk
- Calculator
- Sunny day (for Method 1 only)
- Another person (for Method 1 only)
- An arm-length stick (for Method 2 only)
What to do:
Getting Ready
1. Safety first! This activity requires you to go outdoors, so remember these guidelines for being safe outside: practice social distancing, watch out for cars if crossing streets, and wear sunscreen!
2. The following activities are all about estimating. Think about why you might want to estimate the size or number of something instead of finding the real value. When might an estimate be helpful? What kinds of things have you estimated before?
3. Estimating the height of something tall will require you to measure long distances, too. If you don’t have a really long measuring tape, try this method instead:
- Go outside and find a flat, smooth surface where you can take four big steps.
- Start with your feet together and mark a small line at the top of your feet with the chalk.
- Take one big step forward with one foot and put another chalk mark at the top of that foot. Continue doing that for 4 steps, marking the top of your foot at each step.
- Now measure the distance between each step (they should be similar, but probably not exactly the same). With the help of your calculator, add those numbers together and then divide by four (the numbers of steps you took) to get an average distance of one of your strides. Write down that number for use later.
4. Two different methods for estimating the height of a tall object are listed below. Decide which one you want to try, depending on the space and materials you have available. Or, try both methods and compare them!
Method 1: The Shadow Method
Use ratios to compare your own shadow to a tree’s shadow and calculate the tree’s height.
1. Find a tree or tall pole whose shadow is on a flat surface that you can see and reach (not down a hill, or onto a house or busy street). Tip: do all these measurements at the same time of day because the shadow heights will change depending on where in the sky the sun is.
2. Mark the top of the tree’s shadow on the ground with chalk. (If it is on grass or another soft surface, you could use a twig or other visible object to mark it instead.)
3. Measure the distance from the base of the tree to the top of the tree’s shadow. Use a measuring tape, if you have one long enough. Or, walk that distance using the same strides you measured in the Getting Ready section. Multiply the number of steps you took by the average stride distance you calculated. This number is the height of the tree’s shadow.
4. Now stand up tall so you can see your own shadow on a flat surface. Have another person put a small chalk mark at the top of your shadow and one at the back of your heels. Measure that distance; that number is the height of your shadow.
5. Have the other person measure how tall you are when standing up straight. That number is your height.
6. Now for the math trick: the ratio of the tree’s height to its shadow is going to be the same as the ratio of your height to your shadow. In other words:
Tree’s height = Your height
Tree’s shadow Your shadow
7. Use the rearranged version of that formula below and put in the numbers you measured to find the tree’s height. Use your calculator to help you.
Tree’s height = Your height x Tree’s shadow
Your shadow
Method 2: The Stick Trick
Use the geometry of triangles by creating two similar triangles to help you calculate the tree’s height.
1. Find a tree or tall pole that you can safely walk away from on level ground.
2. You will need a measuring stick or another stick that is the same length as your arm. To measure it, make sure it reaches from your fist or fingers (however you will hold it) all the way to the end of your shoulder. If your stick is too long, start it at your shoulder and mark where it reaches your fingers.
3. Now hold your arm out in front of you and parallel to the ground. Hold onto your stick at the spot you marked so the long portion is up. Your stick and arm should be making two sides of a triangle with a right angle and equal length sides (also called an isosceles right triangle), like the picture below.
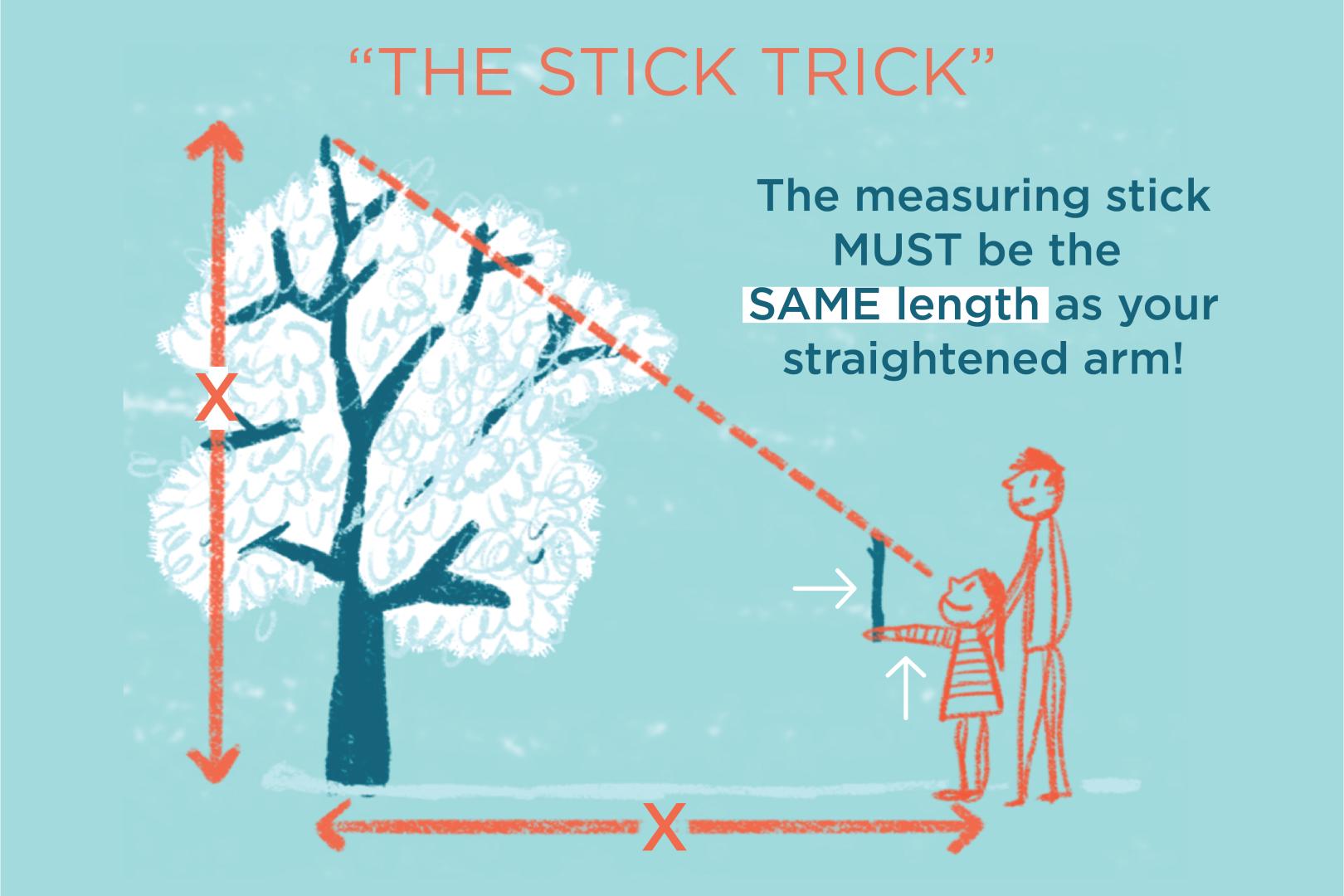
4. Focus your eyes on the top of the stick. The invisible line from your eyes to the top of the stick makes the third side of the triangle!
5. Point that arm toward the tree or pole you want to measure. Walk towards or away from the tree until, from your point of view, you see your fingers (holding the stick) at the base of the tree and the top of the stick at the highest point of the tree.
6. You've now created a second triangle--between yourself, the base of the tree, and the top of the tree--that is similar to the one with your arm and the stick. Once you find that spot, make a small mark where your feet are. You can put the stick away.
7. Now walk from that mark to the base of the tree and count your strides to measure the distance. Try to make your strides about the same as you did in the first section when you calculated your average stride. Don't forget to multiply your number of steps by your average stride distance to find the total distance.
8. Here’s the geometry trick: the big triangle you made with the tree is proportional to the small triangle you made with the stick. That means since the sides of your small triangle (your arm and the stick) are equal to each other, the sides of the big triangle are also equal to each other. In other words, the distance you measured to the tree is about the same as the height of the tree! That number is your tree height estimate.
Reflect
Think and talk about questions like these:
- Estimating is sometimes less accurate than measuring. Where in these methods do you think there could be sources of error—what parts of the process might make it less exact?
- If you used both methods to estimate the same object, how do the results compare? Are they similar or different? If different, by how much?
- Did anyone else try the same method? How did their results compare to yours? Why do you think that is?
What’s happening?
This activity uses the power of estimation to measure a distance that is too hard to measure with normal tools. An estimation is a thoughtful or careful guess based on some information you do know, like counting a few jellybeans in a jar to estimate how many are in the whole jar, or measuring a small portion of something to estimate how big the whole thing is. Estimation can be less accurate than an actual measurement, but it is a helpful tool when getting an accurate number might be too hard, too dangerous, or take too much time.
The shadow method uses a ratio to make this estimate. A ratio shows how much of one thing there is compared to another thing. Here, it allows you to use measurements you can take (like how tall you and your shadow are) to compare to a measurement you can’t take (the height of the tree).
The stick trick uses the geometry of triangles to make this estimate. In geometry, similar triangles are triangles that have the same shape, but one is a larger or smaller version of the other. If triangles are similar, their corresponding angles are equal, and the lengths of the corresponding sides will be proportional too. An isosceles right triangle is one that has a 90-degree angle and two sides of equal length. Here, you used similar isosceles triangles to help you find and measure the distance from the tree that was equal to the tree’s height.

